Algebraical Formulae/Algebraical Identities
Algebra is an important tool to understand the deep concepts of Mathematics and Science. Number Theory, geometry and Analysis etc. are other major departments of Mathematics. It deals with mathematical symbols and the rules for using these symbols. Algebra is the subject of almost all mathematics in a formula. Many things come under algebra, from solving elementary equations to the study of abstract concepts such as the study of groups, rings and fields. The advanced abstract part of algebra is called abstract algebra.
Early algebra is highly essential not only for Mathematics, Science, Engineering but also Medicine and Economics. Early algebra differs from arithmetic in that it uses letters instead of directly using numbers that are either unknown or can hold multiple values.
The application of Algebra is based on solving the equation of variables and constants and deriving the values of variables. The development of algebra resulted in the development of Coordinate Geometry and Calculus, which greatly realized the usefulness of mathematics. The development of Science & Technology is based on various application of Algebra.
The Algebraical Formulae/Algebraical Identities used in Mathematics, Science & Technology and in other fields are as follows:
| 1. |
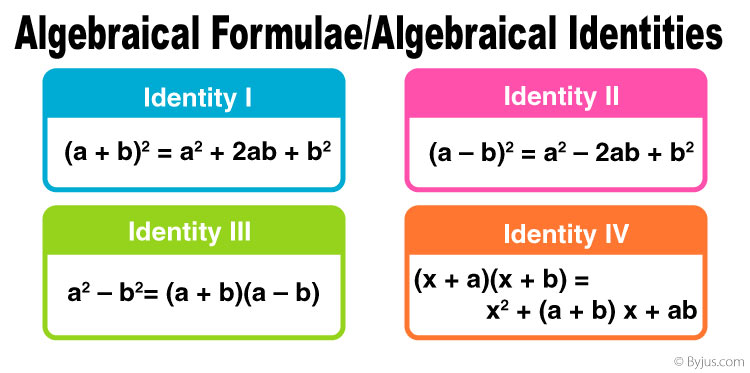
Algebraical Formulae(A + B)2 = A2 + 2AB + B2 [It can be read as A plus B whole square is equal to A Square plus two AB plus B Square] |
| 2. | (A – B)2 = A2 – 2AB + B2
[It can be read as A minus B whole square is equal to A Square minus two AB plus B Square] |
| 3. | (A + B)(A – B) = A2 – B2
[It can be read as A plus B into A minus B is equal to A Square minus B Square] |
| 4. | (A + B)3 = A3 + 3A2B + 3AB2 + B3
[It can be read as A Plus B whole cube is equal to A cube plus three A Square B plus three AB square plus B cube] |
| OR | |
| (A + B)3 = A3 + B3 + 3AB(A+B)
[It can be read as A plus B whole cube is equal to A Cube plus B cube plus 3AB into A plus B] |
|
| 5. | (A – B)3 = A3 – 3A2B + 3AB2 – B3
[It can be read as A minus B whole cube is equal to A cube minus three A Square B plus three AB square minus B cube] |
| OR | |
| (A – B)3 = A3 – B3 – 3AB(A – B)
[It can be read as A minus B whole cube is equal to A cube minus B cube minus 3AB into A minus B] |
|
| 6. | (A + B + C)2 = A2 + B2 + C2 + 2AB + 2BC + 2CA
[It can be read as A plus B plus C whole square is equal to A square plus B square plus C square plus two AB plus two BC plus two CA] |
| 7. | A3 + B3 + C3 – 3ABC = (A + B + C) (A2 + B2 + C2 – AB – BC – CA)
[It can be read as A cube plus B cube plus C cube minus three ABC is equal to A plus B plus C into A square plus B square plus C square minus AB minus BC minus CA] |
| If A + B + C = 0, then A3 + B3 + C3 = 3ABC
[It can be read as if A plus B plus C is equal to zero then A cube plus B cube plus C cube is equal to 3 ABC] |