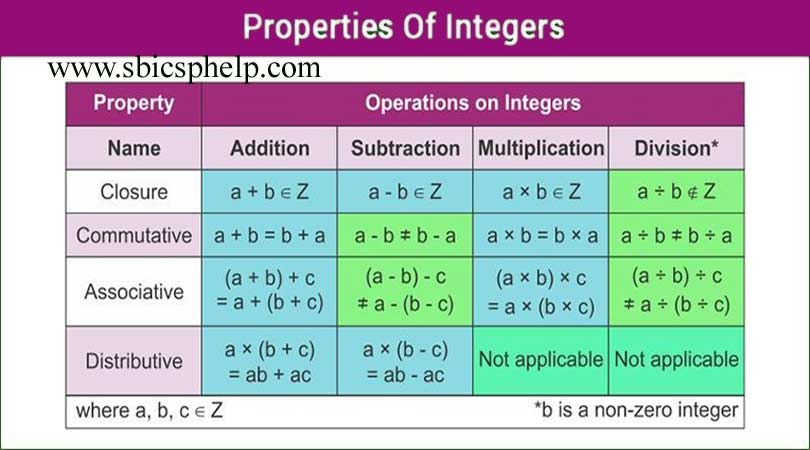
Properties of Integers
- Additive Properties (Properties due to Addition):
a) Closure Property of Addition: The Set of Integers is closed with respect to the operation of Addition. In general, it means that the sum of Integers is always an Integer.
This is known as Closure Property of Addition in the Set of Integers.
For example: 3 and –5 are two Integers, then 3 + (–5) = –2 is also an Integer.
b) Commutative Law of Addition: If a and b are two Integers, then
a + b = b + a.
This is known as Commutative Law of Addition in the Set of Integers.
For example: 3 and –5 are two Integers, then
3 + (–5) = –2
&
–5 + 3 = –2
Hence, it is established that, 3 + (–5) = –5 + 3
c) Associative Law of Addition: If a, b and c are three Integers, then
a + (b + c) = (a + b) + c
This is known as Associative Law of Addition in the Set of Integers.
For example: –3, 5 and –7 are three Integers then
–3 + {5 + (–7)} = –3 – 2
= –5
&
(–3 + 5) + (–7) = 2 + (–7)
= –5
Hence, it is established that, –3 + {5 + (–7)} = (–3 + 5) + (–7)
- Three Reasons Why the Veto Power is So Important
- नाटो क्या है? What is Nato?
- UP TET News Today | यूपी टेट को लेकर बड़ी खबर
- Saarthi app SEBI (Securities and Exchange Board of India)
- Bappi Lahiri Age | Bappi Lahiri We Lost A great singer
- IPL Auction 2022 Players List | IPL Auction 2022 Date
- भारत को UN में मिला बड़ा स्थान – पाकिस्तान के लिए POK खाली करने के लिए भेजा पैगाम
d) Existence of Additive Identity: For every Integer a, there exist a unique an Integer
0 (Zero) such that
0 + a = a + 0 = a
0 (Zero) iscalled the Additive Identity in the Set of Integers For example: Let us consider an Integer 5. Then we have,
0 + (–5) = –5 + 0 = –5
e) Existence of Additive Inverse: For every Integer a, there exist a unique Integer –a such that
a + (–a) = –a + a = 0
a is called the Additive Inverse of –a and vice-versa in the Set of Integers. For example: Let us consider an Integer –5. Then we have,
–5 + 5 = 5 + (–5) = 0
So, –5 is the additive inverse of 5 and vive-versa.
2. Multiplicative Properties (Properties due to Multiplications):
a) Closure Property of Multiplication: The Set of Integers is closed with respect to the operation of Multiplication. In general, it means that the Product of Integers is always an Integer.
This is known as Closure Property of Multiplication in the Set of Integers.
For example: 3 and –5 are two Integers, then 3 × (–5) = –15 is also a
an Integer.
b) Commutative Law of Multiplication: If a and b are two Integers, then
a × b = b × a.
This is known as Commutative Law of Multiplication in the Set of Integers.
For example: 3 and –5 are two Integers, then
3 × (–5) = –15
&
–5 × 3 = –15
Hence, it is established that, 3 × (–5) = –5 × 3
c) Associative Law of Multiplication: If a, b and c are three Integers, then
a × (b × c) = (a × b) × c
This is known as Associative Law of Multiplication in the Set of Integers.
For example: 3, –5 and 7 are three Integers then
3 × (–5 × 7) = 3 × (–35)
= –105
&
{3 × (–5)} × 7 = –15 × 7
= –105
Hence, it is established that, 3 × (–5 × 7) = {3 × (–5)} × 7
d) Existence of Multiplicative Identity: For every Integer a, there exist a unique an Integer 1 (one) such that
a × 1 = 1 × a = a
1 (one) is called the Multiplicative Identity in the Set of Integers
For example: –5 is a an Integer, then
–5 × 1 = –5
&
1 × (–5) = –5
Hence, it is established that, –5 × 1 = 1 × (–5) = –5
Fast Facts
(i) The Set of Integers is closed with respect to the operation of Subtraction. The Difference of two Integers is always an Integer.
Illustrate yourself with suitable example.
(ii) The Set of Integers is not closed with respect to the operation of Division. The Quotient of two Integers is not always a Integer.
Illustrate yourself with suitable example.
properties of the integers
properties of integers worksheet
properties of division of integers class 7
properties of integers under multiplication
properties of subtraction of integers
closure properties of integers
properties of integers ppt
Properties of integers worksheet grade 7
associative property of integers